Understanding Bond Modified Duration in Excel Formulas

Modified duration is a measure of how much a bond’s price will change when the interest rate changes. It is expressed as a percentage of the bond’s current price. For example, if a bond has a modified duration of 5%, it means that if the interest rate goes up by 1%, the bond’s price will go down by 5%. Conversely, if the interest rate goes down by 1%, the bond’s price will go up by 5%. Modified duration is useful for comparing the price sensitivity of different bonds. The higher the modified duration, the more volatile the bond’s price is.

Modified duration is based on another concept called Macaulay duration, which is the average time it takes for a bondholder to get back the money invested in the bond. Macaulay duration is calculated by weighting the time of each cash flow (coupon or principal) by its present value and dividing by the bond’s current price. The longer the time to maturity and the lower the coupon rate, the higher the Macaulay duration. Modified duration is simply the Macaulay duration divided by one plus the yield to maturity divided by the number of coupon payments per year. This adjustment accounts for the effect of compounding on the bond’s price.

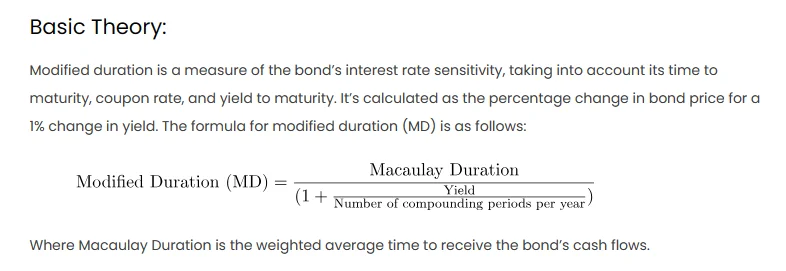
Basic Theory:

Modified duration is a measure of the bond’s interest rate sensitivity, taking into account its time to maturity, coupon rate, and yield to maturity. It’s calculated as the percentage change in bond price for a 1% change in yield. The formula for modified duration (MD) is as follows:

$\text{Modified Duration (MD)} = \frac{\text{Macaulay Duration}}{(1 + \frac{\text{Yield}}{\text{Number of compounding periods per year}})}$

Where Macaulay Duration is the weighted average time to receive the bond’s cash flows.

Procedures:

1. Gather Bond Information: Collect the necessary information about the bond, including its face value, coupon rate, time to maturity, yield to maturity, and the number of compounding periods per year.
2. Calculate Macaulay Duration: Use the following formula in Excel to calculate Macaulay Duration:

$\text{Macaulay Duration} = \sum_{t=1}^{n} \frac{t \times \text{Cash Flow}_t}{(1 + \frac{\text{Yield}}{\text{Number of compounding periods per year}})^{t}}$

1. Calculate Modified Duration: Apply the modified duration formula in Excel:

$\text{Modified Duration (MD)} = \frac{\text{Macaulay Duration}}{(1 + \frac{\text{Yield}}{\text{Number of compounding periods per year}})}$

Comprehensive Explanation:

Consider a 5-year bond with a face value of $1,000, a 6% coupon rate, yielding 5%, and compounded semi-annually.

Macaulay Duration Calculation:

Use Excel to set up a table with columns for the period (t), cash flow, and discounted cash flow.

$\text{Cash Flow}_t = \text{Coupon Payment} + \text{Face Value}$

$\text{Discounted Cash Flow}_t = \frac{\text{Cash Flow}_t}{(1 + \frac{\text{Yield}}{\text{Number of compounding periods per year}})^{t}}$

Sum the discounted cash flows to get Macaulay Duration.

Modified Duration Calculation:

Apply the modified duration formula using the calculated Macaulay Duration.

Real-World Scenario:

Face Value: $1,000
Coupon Rate: 6%
Time to Maturity: 5 years
Yield to Maturity: 5%
Compounding Periods: 2 (semi-annual)

Excel Table:

Period (t)	Cash Flow	Discounted Cash Flow
1	$30	$28.8462
2	$30	$27.5482
10	$30 + $1,000	$826.4463

Macaulay Duration: Sum of the discounted cash flows = $1000.

Modified Duration: Apply the formula:

$\text{Modified Duration (MD)} = \frac{\$1000}{(1 + \frac{0.05}{2})} \approx 9.524$

Result:

The modified duration for the given bond is approximately 9.524.

Other Approaches:

Using Excel Functions: Excel has built-in functions like DURATION and MDURATION that can simplify the calculations.
Solver Add-In: For more complex scenarios, consider using the Solver Add-In in Excel to find the yield that equates the present value of cash flows to the bond price.