Bond Theorem is a general term that refers to different principles or concepts related to bond valuation. Bond valuation is the process of determining the fair price of a bond based on its characteristics and the prevailing market conditions. Some of the common bond theorems are:
- Bond prices are inversely related to interest rate movements. This means that when interest rates increase, bond prices decrease, and vice versa. This is because the present value of the bond’s future cash flows (coupon payments and face value) decreases as the discount rate (interest rate) increases.
- Bond prices are more sensitive to interest rate changes for longer-term bonds than for shorter-term bonds. This is because the longer the time to maturity, the more cash flows are affected by the change in the discount rate. Also, longer-term bonds have lower coupon rates than shorter-term bonds, which means they have less income to offset the price decline.
- Bond prices are more sensitive to interest rate changes for lower-coupon bonds than for higher-coupon bonds. This is because lower-coupon bonds have less income to cushion the price decline when interest rates rise. Also, lower-coupon bonds have a higher duration, which is a measure of the sensitivity of the bond price to interest rate changes.
- Bond prices are more sensitive to interest rate changes when the bond is trading at or near par value than when it is trading at a premium or a discount. This is because the duration of the bond is highest when the bond is trading at par value, and decreases as the bond moves away from par value. A bond trading at a premium has a lower duration than a bond trading at par, and a bond trading at a discount has a lower duration than a bond trading at a premium.
- Bond prices are more sensitive to interest rate changes for callable bonds than for noncallable bonds. This is because callable bonds have an embedded option that allows the issuer to redeem the bond before its maturity date under certain conditions. This option reduces the value of the bond to the bondholder, as the issuer will likely call the bond when interest rates fall and the bond price rises. Therefore, callable bonds have lower prices and higher yields than noncallable bonds with the same characteristics.
Basic Theory:
The Bond Theorem is based on the present value concept, asserting that the value of a bond is the sum of the present
values of its future cash flows. These cash flows include periodic coupon payments and the bond’s face value at maturity.
The present value is calculated using the discount rate, which represents the required rate of return for the investor.
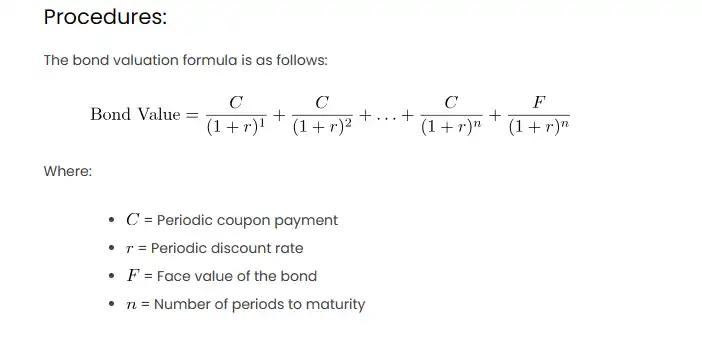
Procedures:
The bond valuation formula is as follows:
![\[ \text{Bond Value} = \frac{C}{{(1 + r)^1}} + \frac{C}{{(1 + r)^2}} + \ldots + \frac{C}{{(1 + r)^n}} + \frac{F}{{(1 + r)^n}} \]](https://excelcalculations.refrigeratordiagrams.com/wp-content/ql-cache/quicklatex.com-ef0f5f9ffcb9c75ed39b9aa1a9d1a2c2_l3.svg "Rendered by QuickLaTeX.com")
Where:
= Periodic coupon payment
= Periodic discount rate
= Face value of the bond
= Number of periods to maturity
Comprehensive Explanation:
Let’s consider a scenario where you have a 5-year bond with a face value of $1,000, a 6% annual coupon rate, and a required
rate of return (discount rate) of 5%.
Excel Setup:
Create an Excel table with the following columns: Period, Coupon Payment, Present Value of Coupon Payment, Present
Value of Face Value, and Total Present Value.
Scenario Calculation:
- Period: 1 to 5 (representing the bond’s life)
- Coupon Payment: $60 (6% of $1,000 face value)
- Discount Rate (r): 5%
Now, use the formula in Excel for each period:
- Present Value of Coupon Payment:
- Present Value of Face Value:
- Total Present Value: Sum of the above two
Results:
Summing up the Total Present Values for all periods gives you the bond value.
Excel Table:
| Period | Coupon Payment | Present Value of Coupon Payment | Present Value of Face Value | Total Present Value |
|---|---|---|---|---|
| 1 | $60 | =60/(1+5%)^1 | =1000/(1+5%)^1 | =Present Value of Coupon Payment + Present Value of Face Value |
| 2 | $60 | =60/(1+5%)^2 | =1000/(1+5%)^2 | =Present Value of Coupon Payment + Present Value of Face Value |
| … | … | … | … | … |
| 5 | $60 | =60/(1+5%)^5 | =1000/(1+5%)^5 | =Present Value of Coupon Payment + Present Value of Face Value |
Calculation:
Summing up the Total Present Values column provides the bond value.
Scenario Result:
The bond value in this scenario is the sum of Total Present Values, indicating the fair market value of the bond
in the given market conditions.
Other Approaches:
- Yield Function in Excel: Utilize the
YIELDfunction in Excel, which directly
calculates the yield of a security based on its coupon rate, face value, settlement date, and maturity date. - IRR Function for YTM: Employ the
IRRfunction in Excel to calculate the Internal
Rate of Return (Yield to Maturity) for the bond’s cash flows.
