Excel Formulas and The Future Value of an Ordinary Annuity Theorem

The Future Value of an Ordinary Annuity (FVOA) is a financial concept that assesses the future worth of a series of equal cash flows received or paid at regular intervals over a specific period. This theorem is fundamental in financial planning and investment analysis. It considers the compounding effect of interest over time, demonstrating how a stream of consistent payments, often made or received at the end of each period, can accumulate into a larger sum in the future. The FVOA provides valuable insights into the potential growth of investments, helping individuals and businesses make informed decisions about savings, retirement planning, and other financial endeavors. Understanding the FVOA is essential for anyone navigating the complexities of financial management, as it illuminates the future value implications of consistent cash flows in the dynamic landscape of compound interest.

Basic Theory:

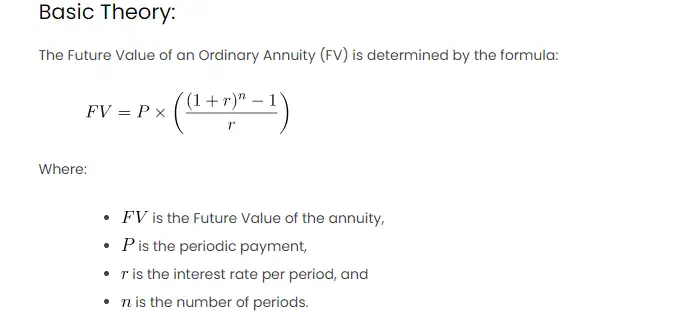
The Future Value of an Ordinary Annuity (FV) is determined by the formula:

$FV = P \times \left( \frac{(1 + r)^n - 1}{r} \right)$

Where:

$FV$ is the Future Value of the annuity,
$P$ is the periodic payment,
$r$ is the interest rate per period, and
$n$ is the number of periods.

Procedures:

To calculate the FV of an ordinary annuity using Microsoft Excel, you can use the following steps:

Identify the Variables:
- $P$ (Periodic payment),
- $r$ (Interest rate per period),
- $n$ (Number of periods).
Enter the Formula: In an Excel cell, input the FVOA formula using cell references for the variables. For example, if is in cell A1, in B1, and in C1, the formula would be:
```
=A1 * ((1 + B1)^C1 - 1) / B1
```
Format Cells: Ensure that cells are formatted correctly. $P$ and $r$ should be positive, and $n$ should be a whole number.
Evaluate the Result: Excel will calculate the FV of the ordinary annuity based on the provided inputs.

Explanation:

Let’s consider a scenario where you invest $1,000 at the end of each year for 5 years at an annual interest rate of 8%.

$P = $1,000$

$r = 8\% \text{ (or } 0.08 \text{)}$

$n = 5$

Scenario:

Period	Payment ( $P$ )	Interest Rate ( $r$ )	Number of Periods ( $n$ )
1	$1,000	8%	5

Calculation:

$FV = $1,000 \times \left( \frac{(1 + 0.08)^5 - 1}{0.08} \right)$

$FV \approx $6,774.05$

In this scenario, the future value of the ordinary annuity after 5 years would be approximately $6,774.05.

Other Approaches:

Excel’s FV Function:Excel provides an FV function that simplifies the calculation. For our scenario, you could use:
```
=FV(B1, C1, 0, -A1)
```
This function calculates the future value of an investment based on periodic, constant payments and a constant interest rate.
Data Table for Sensitivity Analysis:Create a data table to analyze how changes in interest rates or the number of periods affect the future value. This can be done by setting up a range of interest rates or periods and observing the corresponding FV values.