The Future Value of an Annuity Due Theorem in Excel Formula

The Future Value of an Annuity Due is a financial concept that revolves around the idea of investing a series of equal payments at the beginning of each period. Unlike a regular annuity, where payments are made at the end of each period, an annuity due involves payments made at the start of each period. The Future Value of this annuity due represents the total value of these periodic payments at a specified point in the future, taking into account the interest earned on each payment. In essence, it calculates the future worth of a stream of cash flows that begin immediately. This concept is integral to financial planning as it helps individuals and businesses project the future value of investments, aiding in strategic decision-making and ensuring effective management of financial resources over time.

Basic Theory:

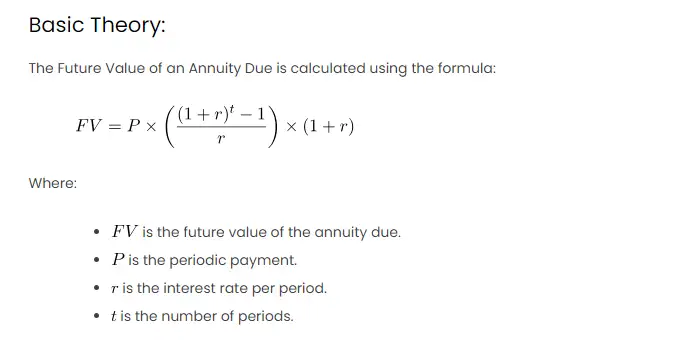
The Future Value of an Annuity Due is calculated using the formula:

$FV = P \times \left( \frac{(1 + r)^t - 1}{r} \right) \times (1 + r)$

Where:

$FV$ is the future value of the annuity due.
$P$ is the periodic payment.
$r$ is the interest rate per period.
$t$ is the number of periods.

Procedures in Microsoft Excel:

Data Entry: Set up an Excel table with columns for “Period,” “Payment (P),” “Interest Rate (r),” and “Future Value (FV).”
Enter Values: Input the relevant data for each column. Ensure consistency in the time unit for the interest rate and the number of periods.
Formula Implementation: In the “Future Value” column, use the formula mentioned above, referencing the corresponding cells for payment, interest rate, and period.
Drag Formula: Drag the formula down for subsequent periods to automatically calculate the future value.

Comprehensive Explanation:

Let’s consider a scenario where an individual invests $1,000 at the beginning of each year for 5 years with an annual interest rate of 8%.

Payment (P): $1,000
Interest Rate (r): 8%
Number of Periods (t): 5

Real-world Scenario Calculation:

$FV = 1000 \times \left( \frac{(1 + 0.08)^5 - 1}{0.08} \right) \times (1 + 0.08)$

Excel Table:

Period	Payment (P)	Interest Rate (r)	Future Value (FV)
1	$1,000	8%	=1000((1+0.08)^1-1)/0.08(1+0.08)

Result:

After performing the calculations, the future value of the annuity due after 5 years is approximately $6,881.50.

Other Approaches:

Built-in Functions: Excel has built-in financial functions like FV that can be used for the Future Value calculation, making the process simpler.
=FV(0.08, 5, -1000, 0, 1)
Data Tables: Utilize Excel’s Data Table feature to analyze various interest rates or payment amounts and observe the impact on the future value.
Graphical Representation: Create a visual representation using Excel charts to better understand the growth pattern of the annuity due over time.